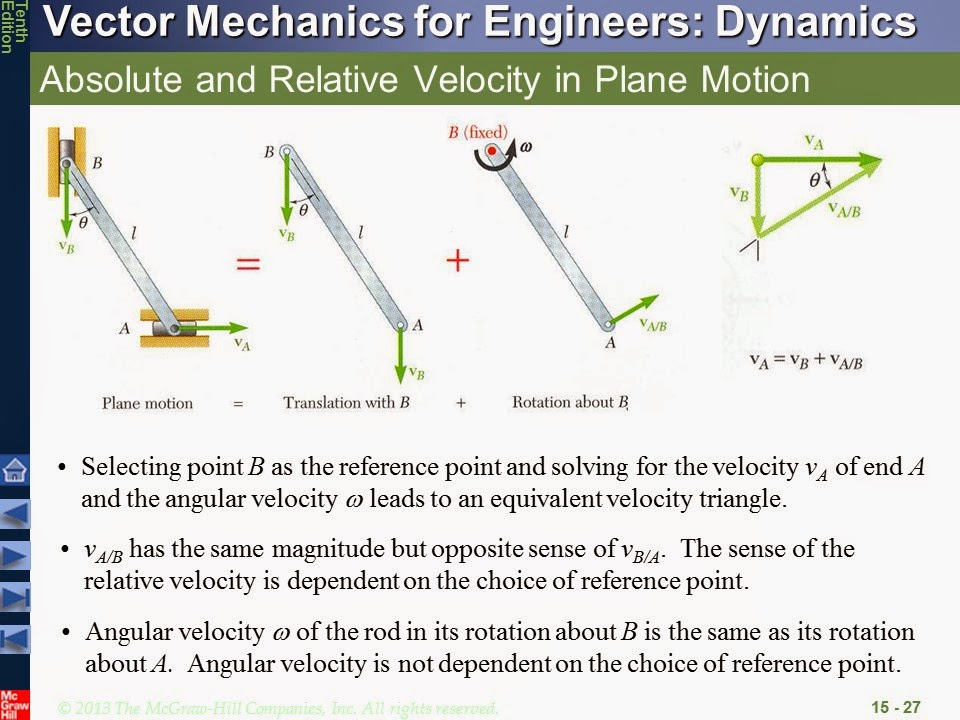
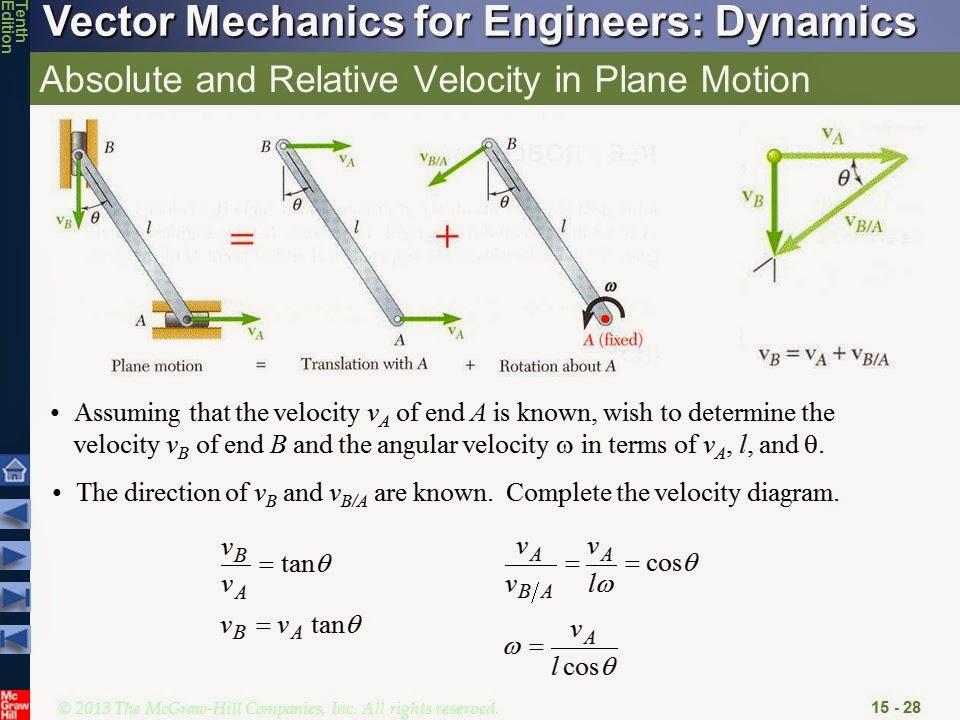
HW: 15.43, 15.54
Lady Bug Revolution:
http://phet.colorado.edu/en/simulation/rotation
1. Constant angular velocity:
Turn on the velocity and acceleration vectors.
Why are the velocity and acceleration vectors pointing the way they are? (why is v tangential, and a normal?)
Vary the position of the bug, and watch the vectors.
How does V vary with r?
How does a vary with r?
Write an equation for acceleration, and velocity as a function of the position of the bug.
~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~
2. How does θ vary with time if the angular velocity is constant?
How does θ vary with time if the angular velocity is increasing? (drag the green arrow to change ω)
Write an equation for θ a function of time, angular velocity, and angular acceleration. Explain where this equation came from.
3. How do the x and y position of the bug vary with time if the angular velocity is constant?
How do the x and y positon of the bug vary with time if the angular velocity is increasing? (drag the green arrow to change ω)
Write an equation for x(t)and y(t) as a function of the position of the bug, and the angular velocity and acceleration.
4. Flip through, and experiment with all of the different graph combinations. Sketch an example of, and label the 3 graphs explaining the relationship between them.
Sketch out and examine each of the 4 “Show Graph” combinations.